
Now the side AD is common in both the triangles \(\Delta ADB\) and \(\Delta ADC\).Īs the line segment AD is the angle bisector of the angle A then it divides the line segment BC into two equal parts BD and CD. Solution: To prove: \(\Delta ADB\) is congruent to the \(\Delta ADC\) Therefore according to the SSS Formula, the two triangles are congruent.Įxample 2: Triangle ABC is an isosceles triangle and the line segment AD is the angle bisector of the angle A. Can you prove that \(\Delta ADB\) is congruent to the \(\Delta ADC\)?
Now the side PQ is common in both the triangles \(\Delta PAQ\) and \(\Delta PBQ\). Two points P and Q, equidistant from the endpoints of the line segment AB. Solution: To prove: \(\Delta PAQ\) is congruent to the \(\Delta PBQ\)
Sss similarity trial#
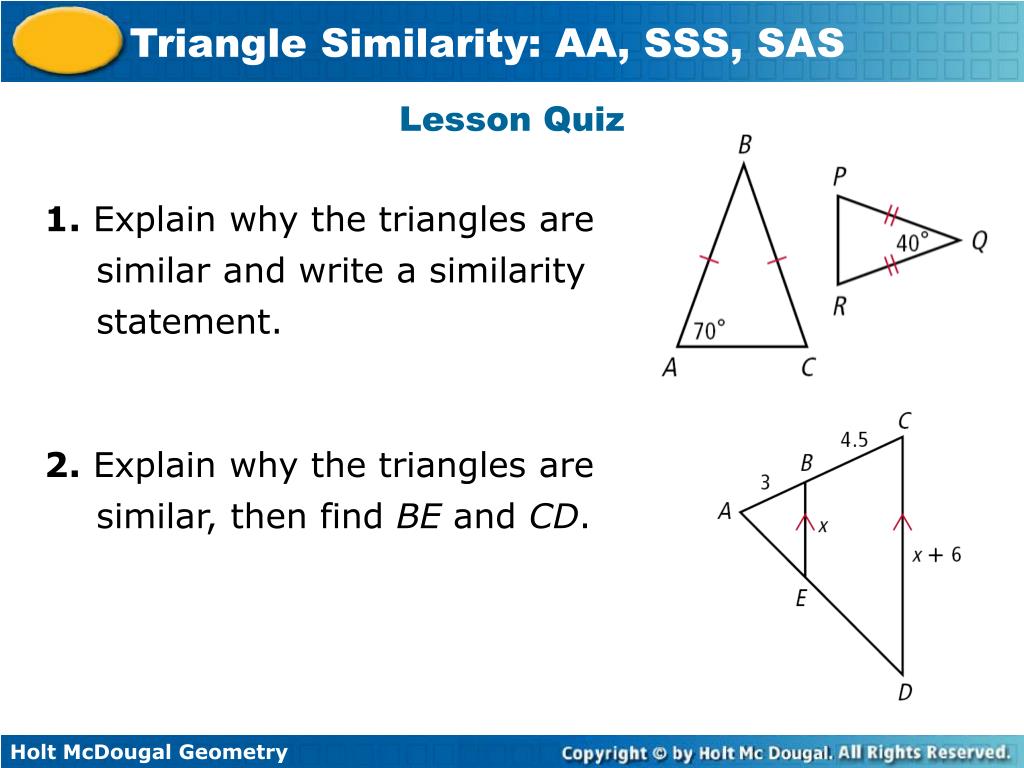
Math will no longer be a tough subject, especially when you understand the concepts through visualizations with Cuemath.īook a Free Trial Class Examples Using SSS FormulaĮxample 1: The two points P and Q are on the opposite sides of the line segment AB. The points P and Q are equidistant from points A and B. Can you prove that \(\Delta PAQ\) is congruent to the \(\Delta PBQ\)?

There are different SSS Triangle formulas used to prove the congruence or similarity between two triangles. Using the SSS Formula, the congruency or similarity of any two triangles can be checked when two sides and the angle between these sides for both the triangles follow the required criterion. Let us understand the desired criterion using the SSS triangle formula using solved examples in the following sections. If two triangles are similar it means that all corresponding angle pairs are equal and all corresponding sides are proportional. However, in order to be sure that the two triangles are similar or congruent, we do not necessarily need to have information about all sides and all angles. If A B P Q B C Q R A C P R, then triangles A B C and P Q R are similar. If two triangles are congruent it means that three sides of one triangle will be (respectively) equal to the three sides of the other and three angles of one triangle will be (respectively) equal to the three angles of the other. If the corresponding sides of two triangles are proportional, then the two triangles are similar.
Sss similarity download#
You can download further information about Similar triangles.Before learning the SSS formula let us recall what are congruence and similarity. Home Side-Side-Side Similarity If the corresponding sides of two triangles are proportional, then the two triangles are similar. If the ratio of the hypotenuse and one side of a right-angled triangle is equal to the ratio of the hypotenuse and one side of another right-angled triangle, then the two triangles are similar. To better organize out content, we have unpublished this concept.
Sss similarity update#
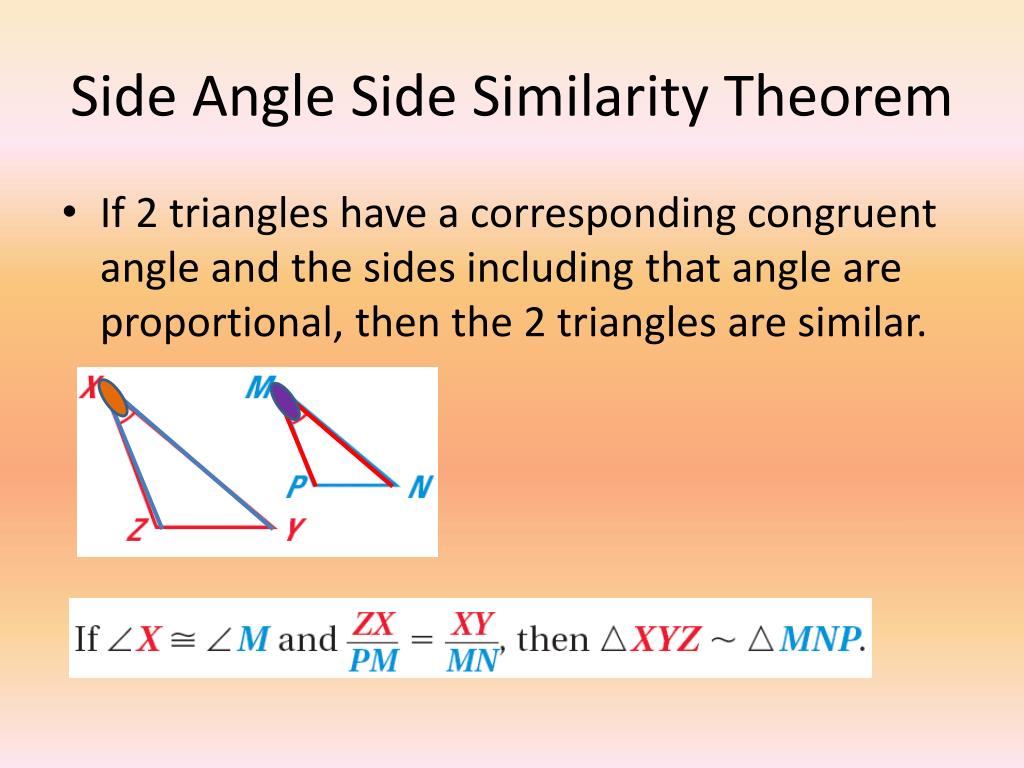
Please update your bookmarks accordingly. Click here to view We have moved all content for this concept to for better organization. We have a new and improved read on this topic. Click Create Assignment to assign this modality to your LMS. If each of the sides of one triangle can be matched up with each of the sides of another so that the ratios of matching sides are equal, then the two triangles are similar. Use the SAS Similarity Theorem to determine if triangles are similar. If the ratio of the lengths of two sides of one triangle is equal to the ratio of the lengths of two sides of another triangle, and the included angles are equal, then the two triangles are similar. Re-stating this fact is not required when using the AAA test in a similarity proof. Get access to the latest SSS Similarity Theorem (in Hindi) prepared with Foundation - Class X course curated by undefined on Unacademy to prepare for the. Because the angle sum of a triangle is always 180°, the third pair of angles will automatically be equal. It is sufficient to prove that only two pairs of angles are respectively equal to each other. If two angles of one triangle are respectively equal to two angles of another triangle, then the two triangles are similar.

There are four similarity tests for triangles. It is helpful if students are also familiar with the tests for congruence.


 0 kommentar(er)
0 kommentar(er)
